The fastball is, as its name implies, the fastest pitch in major league baseball, reaching speeds in excess of 100 MPH, and ideally arriving at the strike zone before the batter can react. Sometimes those fastballs make an unexpected twist that can make or break the outcome of a game. What accounts for differences between pitches? It all comes down to spin speed, spin axis, and the orientation of the ball.
So says Barton Smith, a mechanical and aerospace engineer (and staunch baseball fan) at Utah State University. He studies the complicated physics of how a pitcher's biomechanics can influence the air dynamics of a baseball. Smith presented his findings earlier this week at a meeting of the American Physical Society's Division of Fluid Dynamics in Atlanta, Georgia.
There's some colorful history to the study of baseball pitches, most notably the heated debate in the 1940s and 1950s around whether a curve ball really does curve, or whether it's just a trick of perception. St. Louis Cardinals pitcher Dizzy Dean had this to say to skeptics: "Ball can't curve? Shucks, get behind a tree and I'll hit you with an optical illusion." Dean was right. Curve balls really curve—and we know why in part because of research in the 1950s by Lyman Briggs, a former director of the National Bureau of Standards (now the National Institute of Standards and Technology in Gaithersburg, MD).
Briggs loved baseball. Intrigued by the curve ball question, he enlisted the help of the Washington Senators pitching staff for a series of experiments at the NSB, which boasted a wind tunnel to study aerodynamics. That turned out to be a godsend, because it proved remarkably difficult otherwise to measure all the aspects of a baseball flying through the air—notably its spin, the critical variable in determining how much a curveball curves. Among his findings: a curveball can curve as much as 17-1/2 inches as it flies from the pitcher's mound to home plate.
Spin is a critical element to any type of pitch. "When you throw a baseball, it spins backward," said Smith. "That's a natural thing that happens as a result of your fingers coming off the ball. That makes the ball drop less than it would otherwise." So what accounts for the different behavior of the ball with different pitches? It's got something to do with the seams.
Baseballs are not completely smooth; they have stitching in a figure-eight pattern. Those stitches are bumpy enough to affect the airflow around the baseball as it flies toward home plate after a pitch. It's long been known that the movement of a baseball creates a whirlpool of air around it, commonly known as the Magnus effect. The raised seams churn the air around the ball, creating high-pressure zones in various locations (depending on the type of pitch) that can cause deviations in its trajectory.
A fastball, for instance, creates a high-pressure zone in front of and under the baseball, offsetting gravity's downward pull, so it will fall less than a ball thrown with no spin. But a curveball has a top spin that creates a high-pressure zone on top of the ball, augmenting the gravitational pull and deflecting its trajectory downward. Only the knuckleball is largely unaffected by the Magnus force, because it has no spin. Its trajectory is determined entirely by how the seams affect the turbulent airflow around the baseball.
"The seam acts as kind of a paddle, pushing the air."
"The seam acts as kind of a paddle, pushing the air," said Smith. "If the seam is moving away, it's going to make [the ball] slower. If it's moving in the other direction, it's going to make it faster." In essence, the seams can change the speed (velocity) of the air near the ball's surface, speeding the ball up or slowing the ball down depending on whether said seams are on the top or the bottom of the ball.
There might be variables other than the Magnus effect involved with the so-called "two-seam" fastball, too. As the name implies, two seams are visible with each rotation after the ball is thrown for the two-seam fastball. For a four-seam fastball, the pitcher will grip the ball with two fingers across the space between seams, positioning the edges of his fingers just a bit over the seam. It gets its name from the fact that four seams are visible on the ball with each rotation.
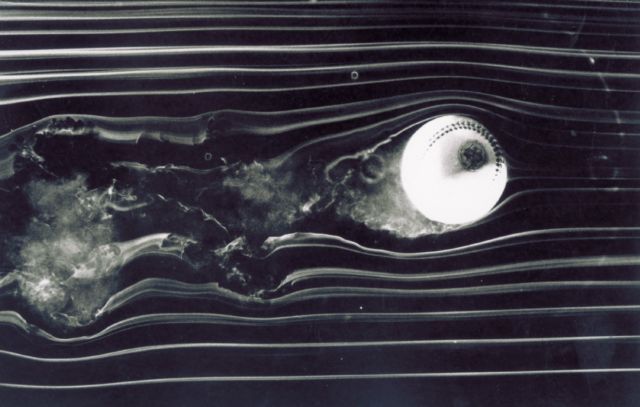
The Utah State University physicists used a pitching machine rather than the typical wind tunnel for their study. Sakib and Smith Two snapshots are taken 20 microseconds apart, soon after a baseball is launched through a smoke-filled chamber. Sakib and Smith
"There is a common notion that a two-seam fastball involves other unique aerodynamics," said Smith, specifically a claim that the transition from laminar (smooth) flow to turbulent flow (dominated by swirls and eddies) of two-seam fastballs is different than four-seam fastballs. That's what he and his graduate student, Nazmus Sakib, decided to investigate. They were especially interested in identifying where, on the ball's surface, the layer of air separates on different kinds of pitches, forming a wake. It's known as the "boundary layer separation."
Smith and Sakib built their own pitching machine in the lab to toss the balls with spins similar to those a real-world baseball pitcher would create. They bought a bucket of Little League baseballs for their experiments and fired them one by one through a smoke-filled chamber, the better to track the tiny movements of smoke particles (and hence changes in the airflow).
Two little red sensors would detect the balls as they moved past, triggering lasers that acted like flash bulbs—the better to capture two successive images of the balls in motion. Then they used a technique called particle image velocimetry to calculate the flow of air at any given spot around the ball. That's how they were able to identify the boundary layer separation.
The result: there is no meaningful aerodynamical difference between a two-seam fastball and a four-seam fastball, according to Smith. Granted, the two pitches have different boundary layer separation as the ball rotates, but both have the same net effect. However, a two-seam fastball can end up having more of a tilted axis of rotation than the four-seam fastball, because one finger leaves the seam before the other as the pitch is thrown. So the ball will move a bit more sideways—a twist in the motion that can be critical.
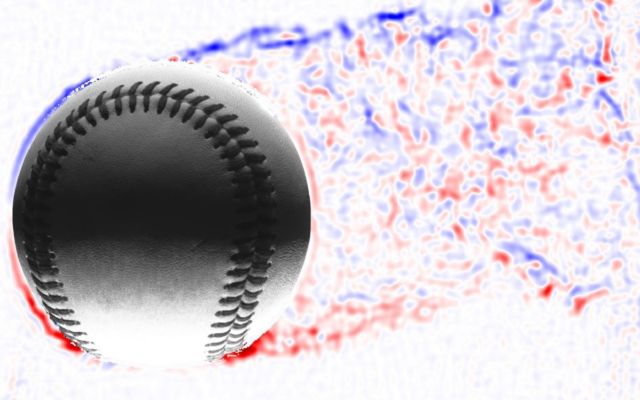
Smith and Sakib also studied the aerodynamics of knuckleballs with their apparatus and found that the flow will separate near the back of the ball any time the seam is located there mid-flight. "This is what happens if you manage to throw a ball that doesn't spin," said Smith. "[Knuckleballs] move rather randomly because of this boundary layer separation. The seam is moving around, so the force on the ball can change from one direction to the other very suddenly and unpredictably."
There's nothing here that will help Major League pitchers improve their skills for the next World Series. These are preliminary results to demonstrate the usefulness of such tools for this kind of study. Smith is hopeful that, longer-term, he might be able to answer the question of why some balls have a larger drag than others—something he says had a significant effect on the number of home runs hit in 2015, 2016, and 2017. Ultimately, "I hope to discover a way to throw a new pitch," he said. "For now, I think it would be helpful to explain to pitchers what their biomechanics does to the fluid dynamics [of the ball]."
[contf] [contfnew] 
Ars Technica
[contfnewc] [contfnewc]







